Logical
operators full truth table
| Input |
Output |
|
|
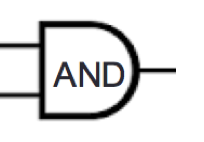
Conjunction |
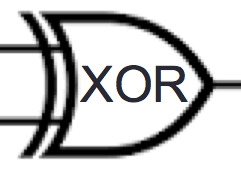
Exclusive or |
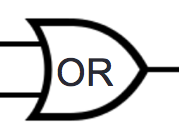
Disjunction |
Conditional |
Biconditional |
| \(p\) |
\(q\) |
\(p \wedge
q\) |
\(p \oplus q\) |
\(p
\vee q\) |
\(p \to
q\) |
\(p
\leftrightarrow q\) |
| \(T\) |
\(T\) |
\(T\) |
\(F\) |
\(T\) |
\(T\) |
\(T\) |
| \(T\) |
\(F\) |
\(F\) |
\(T\) |
\(T\) |
\(F\) |
\(F\) |
| \(F\) |
\(T\) |
\(F\) |
\(T\) |
\(T\) |
\(T\) |
\(F\) |
| \(F\) |
\(F\) |
\(F\) |
\(F\) |
\(F\) |
\(T\) |
\(T\) |
|
|
“\(p\)
and \(q\)” |
“\(p\)
xor \(q\)” |
“\(p\)
or \(q\)” |
“if \(p\) then \(q\)” |
“\(p\)
if and only if \(q\)” |
Logical
operators truth tables
Truth tables: Input-output tables where we use \(T\) for \(1\) and \(F\) for \(0\).
| Input |
Output |
|
|
Conjunction |
Exclusive
or |
Disjunction |
| \(p\) |
\(q\) |
\(p \land
q\) |
\(p \oplus q\) |
\(p
\lor q\) |
| \(T\) |
\(T\) |
\(T\) |
\(F\) |
\(T\) |
| \(T\) |
\(F\) |
\(F\) |
\(T\) |
\(T\) |
| \(F\) |
\(T\) |
\(F\) |
\(T\) |
\(T\) |
| \(F\) |
\(F\) |
\(F\) |
\(F\) |
\(F\) |
|
|
 |
 |
 |
| Input |
Output |
|
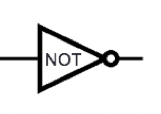
Negation |
| \(p\) |
\(\lnot
p\) |
| \(T\) |
\(F\) |
| \(F\) |
\(T\) |
|
 |



